#18 in a series of articles about the technology behind Bang & Olufsen loudspeakers
Another busy week – so another set of photos…








#18 in a series of articles about the technology behind Bang & Olufsen loudspeakers
Another busy week – so another set of photos…









#17 in a series of articles about the technology behind Bang & Olufsen loudspeakers
This week, instead of talking about what is inside the loudspeakers, let’s talk about what I listen for when sound is coming out of them. Specifically, let’s talk about one spatial aspect of the mix – where instruments and voices are located in two-dimensional space. (This will be a short posting this week, because it includes homework…)
Step 1: Go out and buy a copy of Jennifer Warnes’s album called “Famous Blue Raincoat: The Songs of Leonard Cohen” and play track 2 – “Bird on a Wire”.
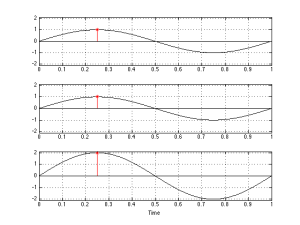
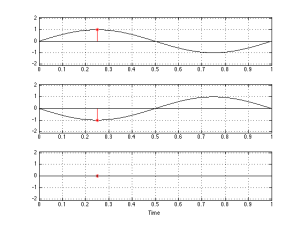
Step 2: Close your eyes and really concentrate on where the various voices and instruments are located in space relative to your loudspeakers. If you hear what I hear, you’ll hear something like what I’ve tried to represent on the map shown in the figure below.
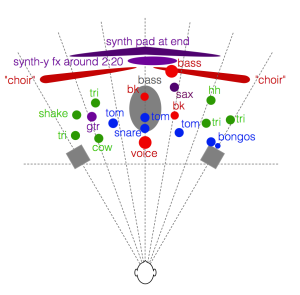
I’ve used some colour coding, just to help keep things straight:
Note that Jennifer sings her own backup vocals, so the “voice”, and the two “bk” (for backup – not Burger King) positions are all her. It also sounds like she’s singing in the “choir” on the left – but it’s hard for me to hear exactly where she is.
Whenever I’m listening to a pair of loudspeakers (or a car audio system, or the behaviour of an upmix algorithm) to determine the spatial properties, I use this map (which I normally keep in my head – not on paper…) to determine how things are behaving. The two big questions I’m trying to answer when considering a map like this revolve around the loudspeakers’ ability to deliver the (1) accuracy and (2) the precision I’m looking for. (Although many marketing claims will use these words interchangeably, they do not mean the same thing.)
The question of accuracy is one of whether the instruments are located in the correct places, both in terms of left and right, but also in terms of distance. For example, the tune starts with a hit on the centre tom-tom, followed immediately by the bigger tom-tom on the left of the mix. If I have to point at that second, deeper-pitched tom-tom – which direction am I pointing in? Is it far enough left-of-centre, but not hard over in the left loudspeaker? (This will be determined by how well the loudspeakers’ signals are matched at the listening position, as well as the location of the listening position.) Secondly, how far away does it sound, relative to other sound sources in the mix? (This will be influenced primarily by the mix itself.) Finally, how far away does it sound from the listening position in the room? (This will be influenced not only by the mix, but by the directivity of the loudspeakers and the strength of sidewall reflections in the listening room. I talked about that in another blog posting once-upon-a-time.)
The question of precision can be thought of as a question of the size of the image. Is it a pin-point in space (both left/right and in distance)? Or is a cloud – a fuzzy location with indistinct edges? Typically, this characteristic is determined by the mix (for example, whether the panning was done using amplitude or delay differences between the two audio channels), but also by the loudspeaker matching across the frequency range and their directivity. For example, one of the experiments that we did here at B&O some years ago showed that a difference as small as 3 degrees in the phase response matching of a pair of loudspeakers could cause a centrally-located phantom image to lose precision and start to become fuzzy.
You might note that I used the term “two-dimensional space” in the beginning of this posting. In my head, the two dimensions are (1) angle to the source and (2) distance to the source. I don’t think in X-Y cartesian terms, but Polar terms.
An important thing to mention before I wrap up is that this aspect of a loudspeaker’s performance (accuracy and precision of phantom imaging) is only one quality of many. Of course, if you’re not sitting in the sweet spot, none of this can be heard, so it doesn’t matter. Also, if your loudspeakers are not positioned “correctly” (±30 degrees of centre and equidistant from the listening position) then none of this can be heard, so it doesn’t matter. And so on and so on. The point I’m trying to make here is that phantom image representation is only one of the many things to listen for, not only in a recording but also when evaluating loudspeakers.
#16 in a series of articles about the technology behind Bang & Olufsen loudspeakers
Take a balloon and blow it up. It will look something like the drawing in the centre of Figure 1.
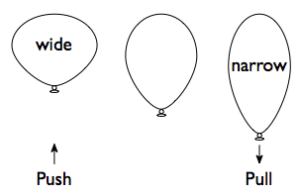
If you put your hands on the top and bottom of the ballon and compress them, you’ll make the balloon shorter, but you’ll also make it wider (as is shown on the left side of Figure 1). This is because the air inside the balloon is under a higher pressure when you squeeze your hands together, and that pressure pushes harder on the parts of the balloon that aren’t being held in by your hands.
If, instead, you grab the top and bottom of the balloon and stretch them further apart, you’ll make the balloon narrower (as is shown on the right side of Figure 1). This is because you’ve created more space inside the balloon, thus lowering the internal pressure pushing out on its walls. The lower the pressure, the less the air pushes outwards, so the balloon collapses.
What we’ve described here is basically the same as what happens to a sealed loudspeaker cabinet (if you’re not careful when you do its mechanical design). However, instead of your hands squeezing a balloon (a sealed “cabinet” of air), we use the signal from a power amplifier to pull the woofer into the cabinet. This increases the pressure inside the cabinet, since it’s sealed and there’s nowhere for the air to go. As a result, the trapped air tries to push outwards on the sides of the cabinet. If the walls of the cabinet are thin, then the cabinet itself will act like the balloon and expand.
Similarly, if you put a positive signal on the power amplifier, you’ll move the woofer out of the cabinet, reducing the air pressure inside it and “sucking” the sidewalls of the loudspeaker in (if they are so thin that they can move).
Generally speaking, you want a loudspeaker to behave as a piston in a baffle (like this). This means (in this case) that you want the woofer to move in and out of a loudspeaker cabinet and you don’t want the cabinet itself to move at all. (We’ll talk later about why this is a bad thing.)
There are a number of different ways to do this. One way is to make your loudspeaker cabinet out of something very, very, very stiff (and probably, as a result, very, very, very heavy). For example, we make our prototypes out of 22 mm or 25 mm thick MDF (and sometimes we use two sheets of it, glued back to back, to make sure that things are stiff enough). Most of our loudspeakers like the BeoLab 9, for this week’s example, have enclosures that are made of plastic, so we use some different methods to achieve the stiffness and rigidity we need to ensure that our enclosures are not singing along with the drivers.

The first step in ensuring that the walls of the loudspeaker cabinet are stiff enough is to make them thick enough. How thick is “enough” depends on the loudspeaker itself. A subwoofer with a small enclosure volume will have to withstand more internal air pressure than a midrange in a larger enclosure. Of course, we can’t simply make the walls of the cabinet out of overly thick plastic, since that would not only be an unnecessary waste of materials, it would increase the weight of the loudspeaker (a consideration for shipping) and the cooling time of the plastic when it’s in production (a consideration for the production line timing and costs).
A second way to make the sidewalls stiffer is to use ribs – usually on the inside of the cabinet. These are moulded as part of the sidewall itself – they aren’t just glued on to the inside surface of the cabinet. If you take a look at Figure 3, you can see the ribs on the inside of the sidewalls of the BeoLab 9. These run diagonally and vertically in the case of this loudspeaker – but they’re not just randomly placed inside the loudspeaker. They have been strategically placed using simulations in the early development stages, and measurements of the early prototypes. These measurements are done using very small accelerometers glued to the sides of the loudspeakers and monitoring their outputs while playing signals through the loudspeaker drivers. (In case you’re wondering, the depth of the ribs is about 22 – 25 mm, depending on where you measure.)

A third tactic is to use a plastic that is stiffened by adding things to it. The usual method is to use fibre-reinforced plastic. The fibres in the plastic help give it a structural strength that you can’t get from just plastic alone.
A fourth possibility is to create a laminate material where you build up the enclosure using layers of different materials (or layers of the same material with different structural composition). This increases stiffness in the same way that a sheet of plywood is stiffer than a sheet of wood.
So, in the case of the BeoLab 9 (as with many of our other loudspeakers), three of these tactics were used. The plastic is thick enough, it has strengthening ribs on the inside, and it is a laminate (if you slice it open, you’ll see that it is a layer of foamed plastic, sandwiched between “skin” layers of solid plastic).
However, when they (I wasn’t part of the BeoLab 9 development team – I was still working in the Automotive Department at the time) got to the last stages of the development, it became obvious that there was a problem. There was an audible resonance caused by the woofer. Some digging around resulted in the finding out that the sides of the cabinet were moving too much. In essence, the problem was almost exactly as I described with the balloon in Figure 1.
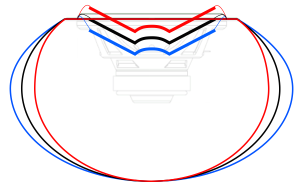
As I tried to show in Figure 4, when the woofer moved inwards, it pushed the sidewalls of the loudspeaker outwards (shown with the blue lines). When the woofer moved outwards, it sucked the sidewalls inwards (the red lines). (I’m over-simplifying here, but not enough to start a fight.)
However, as I said, this was discovered rather late in the development process. The problem had to be fixed, but the question was how to do it without starting from scratch and creating new moulding tools for making the plastic enclosure. The solution was to use the sidewalls to reinforce each other. Since the movement of the opposite sides was in opposite directions (i.e. the left and right sides of the loudspeaker either wanted to move apart or together at any given moment) they could be braced by connecting them with a bridge. Take a look again at Figure 3. You’ll see a metal rod that goes straight across the middle of the woofer enclosure. You can see it just above the back of the woofer in Figure 5 as well.

That piece became known in the acoustics department as the “dog bone” because its final version had the basic shape of a cartoon dog bone. The end result is that the rod is included in the BeoLab 9 construction to prevent the sidewalls of the woofer cabinet from moving when you’re playing loudly.
Here’s another photo showing the internal PCB with the amplifier and filters – not because it’s relevant to this discussion, but just because it might be interesting…

So, we’ve seen how we get rid of parts of the loudspeaker moving when they shouldn’t. The question is “why is it a big deal?” Well, it’s a little like Sir Robert Helpmann’s comment about the difficulties in choreographing a nude ballet – the problem is that some parts of the body keep moving when the other parts have stopped.
In theory, a loudspeaker should behave the same at all frequencies (that statement can mean a lot of different things – and I am happy to argue that it is both true and false, depending. So don’t try to start a fight with me on that one, and please don’t mis-quote me and say that I’m contradicting Siegfried Linkwitz or anyone else by taking that statement out of context on a hi-fi forum somewhere else…) As I mentioned in a previous posting, we like to pretend that a loudspeaker is just a moving piston in an infinite baffle, since that behaves pretty well. Of course, no one actually believes that this model is true – but it’s a comforting ideal.
Take a look at the shape of the blue and red versions of the loudspeaker cross-section in Figure 4, above. You might notice that, when the woofer goes outwards, the cabinet goes inwards. When the woofer goes inwards, the cabinet goes outwards. (this is an oversimplification of the truth, but let’s go with it for now). This means that, from the point of view of the air pressure radiating from the entire loudspeaker, the woofer goes positive and the cabinet goes negative at the same time. So, the sound pressure radiating off the cabinet cancels the sound pressure radiating off the woofer as can be seen in an example of two opposite-polarity sources causing destructive interference as in the animation below. (Note the intersection of the red and green curves where you will hear no sound at all…)
Not only that, but the cabinet as a sound source has a very different directivity than the woofer by itself. So, the result is… complicated. The truth is even more complicated, since the cabinet will not behave as nicely as this – it will resonate better at some frequencies than others, making some notes “bloom” – they’ll appear to be louder (or quieter, depending on where you are and how the room interacts with the loudspeaker) and they might even appear to come from a different direction.
So, the moral of the story here is that you want all parts of the loudspeaker to not be moving when the parts that are supposed to be moving are doing so.
And, before you go and listen to your loudspeakers and look for “blooming” and blaming it on panel resonances or vibrating cabinets, don’t forget that your room modes are more likely a primary source of mis-behaviour when it comes to some bass notes sounding different from the others. However, if you have problems with your room acoustics, we can’t fix that with ribs and dogbones – unless you also bring in a large dog. (I know, I know – the linked paper is about humans – but it does make a mention of animals in the abstract…)
#15 in a series of articles about the technology behind Bang & Olufsen loudspeakers
Before we start talking about curves and corners, let’s have a quick review on the concept of interference. At its most fundamental level, sound is just a relatively small, relatively fast change in barometric pressure. If the instantaneous pressure is higher than average (which happens to be the same as the pressure inside your head), then your eardrum is pushed into your head. If the instantaneous pressure is lower than average, then your eardrum is pulled out of your head. When your eardrum moves in and out, you hear sound.
One way to create a high pressure is to take a loudspeaker driver and push it outwards. In order to create a low pressure, we pull it inwards, as is shown in the animation below. The red thing is a piston which is basically the way we like to pretend a loudspeaker driver (like a tweeter) behaves. The grey thing is a very, very wide loudspeaker cabinet. The red semicircles show the high pressure zones that expand outwards from the front of the loudspeaker. The green semicircles show the low pressure zones.
If you have two sound sources, their pressure differences (relative to the average pressure) add. So, if you have two high pressures arriving at your eardrum, it will be pushed farther into your head than if only one of them arrived at your ear. Similarly, if you get two low pressures arriving at your eardrum, it will be pulled further out of your head than if only one of them was present. HOWEVER, if you have a high pressure and a low pressure arriving at the same time in the same place (for example, at your eardrum) then they cancel each other and, if they have the same magnitude, your eardrum won’t move at all and you won’t hear anything. (This is how noise-cancelling headphones work. The sound from the headphones is in theory identical to the sound coming to your ears from outside the headphones, except that it’s opposite in polarity, so the sum of the two sounds is nothing.)
Keep all that in mind as you read on…
It should not come as a surprise that a sound wave will bounce off a hard surface like a flat concrete wall. The question is “why?” The answer to this question can be complicated – but the simple version is that the molecules in a concrete wall are harder to move than the molecules in air – so we have a change in acoustic impedance. This is essentially a measure of how easily the molecules in the substance are moved by a sound wave… sort of… (Let’s leave it at that, since we really don’t need this article to be a thorough discussion of acoustic impedance.)
The interesting thing is that an acoustic wave will be reflected off any change in impedance. So, you don’t have to be going from a low impedance to a high impedance (as in the case of a sound wave trying to move from air into concrete). It will also reflect on a boundary where you change from a high to a low impedance (for example, a sound wave in concrete trying to get out into air – in this case, the sound wave will bounce off the surface of the concrete and move back into it rather than “leak” out into the surrounding air.).
Imagine yourself standing in a long tunnel. You clap your hands, and the sound wave travels down the length of the tunnel until it reaches the end – what happens then? Well, the answer is “two things”. Some of the sound leaks out of the tunnel. However, since the air inside the tunnel has a higher acoustic impedance than the air outside the tunnel (because the sound is freer to go where it wants on the outside), the end of the tunnel is a boundary where there is a change in acoustic impedance. And, as we saw in the last paragraph, this means that we will get a reflection. So, even though the end of the tunnel is open, it reflects your hand clap back into the tunnel. So, some sound leaks out and some reflects. (One way to really experience this is to notice your ears pop when you enter a long tunnel on a fast-moving train. When you first enter the tunnel, your ears pop because of the sudden change in pressure. Some time later, you might notice that your ears pop again. This is because the high-pressure wave front that the train made when it entered the tunnel travelled to the opposite end of the tunnel, bounced back and hit you again.)
If you didn’t know that the second sound was a reflection off the end of the tunnel (for example, you didn’t hear the first hand clap because you were wearing earplugs) you might think that it was a direct sound from someone down at the far end of the tunnel. So, if you’re not the person doing the clapping, but you’re in the tunnel with that person, you get two sounds – the direct sound and the reflection.
There is another, less obvious case where you have a change in acoustic impedance. This is when you have a sound wave travelling along next to the surface of something, and the surface ends. For example, if, in the animation at the top of this page, the surface of the loudspeaker cabinet was not as wide, there would be a corner where the face of the loudspeaker meets its side. At that corner, the acoustic wave front “sees” a change in acoustic impedance. Consequently, there is something like a reflection that starts at the corner. in essence, the corner of the loudspeaker is a boundary that radiates like a second sound source (just like the end of the tunnel in the example above).
So, if we modify the animation at the top of the page to include a narrower cabinet, the result would be something like the animation below.
As you can see there, the corner of the loudspeaker becomes a second source that radiates its own sound waves after the original, direct sound hits it.
This effect is called acoustic diffraction and it has some significant implications on the sound of a loudspeaker. This is directly because of the interference (see above…) between the direct sound from the loudspeaker driver and the secondary sound source caused by the corner.
Remember we saw above in Fig.1 that, when you have two high pressure zones that meet each other, you get more pressure than either one of them alone. Now take a look at the animation above and look for the places where the black curves from the two “sources” intersect. This is where you’ll get an increase in pressure, and therefore more energy than just the direct sound by itself. As you can see in Fig. 3, below, this means that you have an angle off-axis to the front of the loudspeaker where the signal is louder than it is directly on-axis. Of course, this also means that there will be some angles where you hear less (because the secondary wavefront from the corner cancels the direct sound) – these are where the red and green curves (the high and low pressure zones) intersect.
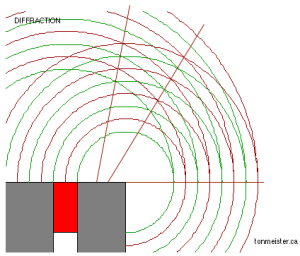
As you can see in Fig 3a, there are different angles where the high pressures add to give you an even higher pressure (notice that the low pressures also add to give you an even lower pressure. The result is that, along those lines, you get constructive interference and therefore the sound is louder than it is elsewhere. I’ve only shown three such angles in this diagram – there are more. You might note as well that the origin of the high pressure lobes is not the centre of the loudspeaker driver (the piston shown in red). It’s somewhere between the primary and secondary sources (in this case, the loudspeaker driver and the corner).
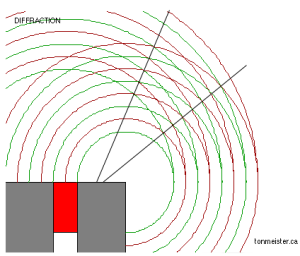
Fig 3b shows two angles where the high and the low pressures overlap, causing destructive interference and therefore cancellation. Therefore, the sound is quieter along those lines than it is elsewhere.
What does all of this mean in the real world?
Well, as you’ve probably already guessed, the first conclusion is that building a loudspeaker that has sharp corners is probably a bad idea. For example, if you wanted to build a loudspeaker, and you just put a tweeter on the front and made sharp right angles where the sides meet the front, you will have a problem with diffraction off those corners. As you can see in Figure 3, you will get a boost in the signal at some angles off-axis to the front of the loudspeaker, and some cancellation at other angles. The amount by which the signal will be boosted, the angles where you’ll have the effects, and the frequencies where you’ll have the problems are all dependent on the specific dimensions of the device. For example, the further away the loudspeaker’s corner from the driver, the lower the frequency that will be affected.
Let’s take a real-world example. The very first version prototype of the BeoLab 5 was really just a “normal” three-way loudspeaker that was used to test the ABC algorithm, So, there was a woofer in a cabinet with a microphone for the ABC development, but on top was just a small cabinet with a midrange driver and a tweeter, as you can see in Figures 4 and 5.



Figure 6 shows the “conventional” tweeter cabinet version of one of the BeoLab 5 prototypes which was placed on top of the white woofer cabinet shown in Figure 4 when the Acoustic Lens assembly was removed. As you can see, this is an example of how-not-to-make-a-loudspeaker (if you’re worried about diffraction). We have a tweeter in a flat surface and (some sharp-ish corners at the sides of the loudspeaker face). The result of this is that we have exactly the same problem shown in Figure 3a and 3b, above. We can see this in the measurement of the horizontal directivity of the loudspeaker, shown in Figure 7, below.

It may be a little difficult to read this plot, so I’ll explain a little. The entire plot has been normalised to the on-axis magnitude response of the tweeter. In other words, the measurement doesn’t show the response of the tweeter – it shows how the response changes as you move around the loudspeaker in the horizontal plane. The X-axis is the frequency of the signal in Hz, ranging from 1.8 kHz to 20 kHz. The Y-axis is the horizontal angle of radiation of the loudspeaker where 0° is directly on-axis, in front the tweeter. The lines in the plot can be thought of as a kind of topographical map with a difference of 0.5 dB per contour. So, if you think of a straight “ridge” in the plot along the 0° line in the middle, the plot generally falls off (in other words, the signal is quieter) as you move around to the side and back of the loudspeaker. You can see that, at the high frequencies, the lines are closer together which means that you lose more level at high frequencies than at low frequencies as you come around to the side of the loudspeaker. This is traditionally called loudspeaker “beaming”. The interesting thing to look at are the four red oval areas. The larger ones are centred around 3.2 kHz and ±40°. The smaller ones are up at about 7.5 kHz and about ±15°. Because they’re in red, this means that they are louder than the on-axis response, so they are peaks in the topographical map. These peaks are the direct result of diffraction off the edge of the loudspeaker cabinet. I count 4 red contour lines at the lower frequency peak, which means that we have a beam that is about 2 dB (remember, 0.5 dB per line * 4 lines) louder around 3 kHz at 40° off-axis to the loudspeaker.
This cabinet was built compare the directivity of a normal box-shaped loudspeaker to one with an Acoustic Lens. A close-up of the lens used for this comparison is shown below in Figure 8.

You’ll note in Figure 8 that the Acoustic Lens is slightly different from the final version (hence the “first-generation” qualifier) . This version also suffered from diffraction artefacts caused by the sharp edges where the face of the lens structure meets its side. This was corrected in the second generation version shown below in Figure 9.

Notice that the second-generation lens has curved transitions from face to side to reduce the diffraction problem. This curvature was eventually extended to wrap around the entire structure as can be seen in the photo of the final BeoLab 5 tweeter lens in Figure 10, below.

You may notice that the difference in these two designs was that the original one had sharp corners on the sides. The diffraction effects of these corners were easily visible in the first directivity measurements of the Lens, so the second prototype with the curved transition from front to side was made to eliminate this problem. The directivity measurement of the prototype shown in Figure 9 is seen below in Figure 11.
You’ll see in Figure 11 that there are two significant differences between the directivity of a tweeter in the prototype Acoustic Lens and a conventional cabinet (shown in Figure 7). The first difference is that the beaming effect (seen as a convergence of the contour lines at the high frequencies in Figure 7) does not happen with the lens. The contour lines are much more parallel resulting in a behaviour known as “constant directivity”. This is a way of saying that the loudspeaker has a directivity that is the roughly the same throughout its entire frequency range (rather than beaming in the high end).
The second difference is that the peaks in the 3 kHz and 8 kHz areas, seen in Figure 7, are gone. This is because there are no corners at the edge of the loudspeaker cabinet to cause diffraction. You may note a peak in the magnitude responses off-axis above 15 kHz. We actually don’t know what causes this, however, since it is so high in frequency and only +1 to +1.5 dB, and since this is still only a prototype, it wasn’t really considered to be a significant issue.
So, I’ve killed two birds with one stone in this article (or “two flies with one smack” as they say in Denmark). On the one hand, we’ve seen that, if you’re worried about the directivity and/or the off-axis response of your loudspeaker (I know, the latter is a sub-set of the former…) sticking a tweeter (or a midrange, or a woofer, depending on dimensions and frequency ranges) on the front of a rectangular box is probably a really bad idea. (On the other hand, it’s a pretty easy, and therefore cheap, way to build a speaker, which is why such a design is so popular I guess…) And, on the other hand, we’ve seen one of the characteristics of Acoustic Lenses – being a more constant directivity than a tweeter-on-a-box. The fact that the tweeter mounted in an Acoustic Lens had less diffraction is not because of the Lens geometry in particular, but because of the shaping of its surroundings as part of the development process of BeoLab 5.
There are more stories like this one. For example, if you look carefully at the “plates” of the BeoLab 5 and the prototype in Figure 4 (the part the tweeter and midrange drivers are mounted in), you might notice that the prototype plates are flat, whereas the BeoLab 5 plates curve downwards. This is not because someone thought the curve would look pretty. This was because the circular edge of the prototype plates also caused diffraction, resulting in a higher-level lobe in the vertical plane. Sloping the plates downwards puts their sharp edges in the “shadow” of the plates themselves, reducing the diffraction effects. So, you can see that diffraction and its effects on directivity is one of the other issues that we worry about when we’re building a loudspeaker.
#14 in a series of articles about the technology behind Bang & Olufsen loudspeakers
Rather than talk about technologies inside B&O equipment, this week I’ll try to go through a couple of strategies on how to properly calibrate the main channels in a surround system – and how to do it improperly, but make it sound better for your friends. I’ll use the example of a BeoPlay V1, a BeoVision 11 or a BeoSystem 4 as the heart of the system – but the basic concepts are the same for any other surround processor.
The first step in setting up any surround sound system is the correct placement of your loudspeakers. There are two standard configuration recommendations. The first is from the International Telecommunications Union, in a document called Recommendation ITU-R BS.775-2 – Multichannel stereophonic sound system with and without accompanying picture (available as a PDF file from the ITU here). The second is called Recommendations for Surround Sound Production from the Producers and Engineers Wing of the Recording Academy of the National Academy of Recording Arts and Sciences (or NARAS – better known as the people that bring you the Grammys). Tha recommendation can be downloaded as a PDF file from here).
The short versions of these two recommendations are as follows:
The ITU standard configuration is the one people who do research into multichannel audio use for their experiments. It’s also the one we use at Bang & Olufsen when we’re testing our loudspeakers in the Acoustics Department or tuning the parameters in the TrueImage upmixing algorithm. The nice thing about this configuration is that it matches a surround sound system for someone who sits on a sofa placed against a wall, and has their surround loudspeakers adjacent to the same wall.
In a perfect loudspeaker configuration, all of your loudspeakers are the same distance from the listening position. They have all been calibrated to have the same loudness at the listening position. Also, they are all large, full-range loudspeakers (and therefore, you do not need a subwoofer).
The Centre Front loudspeaker should be in the centre, at the front (we’ll call that 0°). The Left Front and Right Front loudspeakers should be at ±30° relative to that angle. The Left and Right Surround loudspeakers should be located symmetrically at an angle of between ±100° and ±120°.
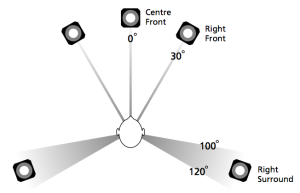
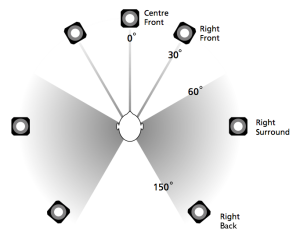
The ITU-775 document doesn’t specifically state the standard configuration for a 7-channel system, but it does provide a recommendation for a 5-channel system that uses 7 loudspeakers (in cases where you have a larger system and you use two loudspeakers per surround channel). However, the recommendation is still a pretty good recommendation for a 7-channel setup. (This also makes sense, since, if you have 7 loudspeakers, you may occasionally like to use them as a 5-channel system without having to place extra loudspeakers in your room.) If you dig around, you’ll see that this also fits the typical setups used in re-recording studios for doing 7-channel mixes and mastering for Blu-ray releases of films. A good example of this is Tron Legacy, which was produced using a system very much like the one shown below – with matching loudspeakers at 0°, ±30°, ±90° and ±150°. (this also makes sense from a radially symmetry perspective, since, ignoring the centre channel, you have equal loudspeaker spacings of 60°.
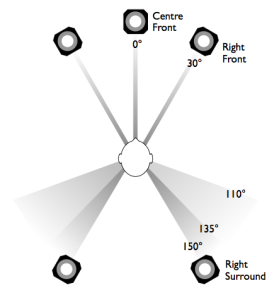
The NARAS recommendation is a little different, although the people that wrote it were aware of the ITU recommendation (which came first…), so they made sure that their version didn’t contradict the existing standard. Their version uses the same layout for the front three loudspeakers, but suggests that the surround loudspeakers be a little further back – within the ±110° to ±150° angle, with an “optimal range” of ±135° to ±150°.
Like the ITU standard, the NARAS document also recommends that all loudspeakers be the same type of full-range loudspeakers, all the same distance from the listening position, all level-adjusted to be the same at the listening position.
Both the ITU and the NARAS standards are really designed by and for people who work and live in recording studios or run perceptual experiments involving multichannel audio. This means that they have one chair and no friends – at least when they watch movies and listen to music… However, if you have a sofa and friends, then you will start having some questions – or at least some doubts.
For example, if you are “normal” (whatever that might mean) but a little careful about your surround sound setup, you probably have something that looks like the drawing below.
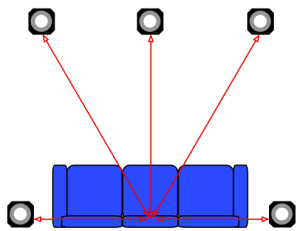
What happens if we were to calibrate this system “perfectly” using the centre of the sofa as our reference “sweet spot” as shown in Figure 4? We’d apply a delay to the Centre Front loudspeaker to make the time of arrival of its signals match the Left Front and Right Front loudspeakers (usually done by setting the Speaker Distance). We’d also apply a delay to the surround loudspeakers to do the same. We’d also probably drop the levels of the centre and surround loudspeakers to match the Left Front and Right Front signals (because they’re closer, and therefore louder).
However, let’s think about what happens if you sit on the left side of that sofa? Now, the Left Surround loudspeaker is very close to your left ear – and that has some serious implications on your experience. Firstly, since sound pressure doubles with every halving of distance, (assuming that this diagram is to scale) then sitting on the left side of the sofa means that you’ll get roughly a 6 dB boost (possibly more, if you’re leaning…) in the signal from that one loudspeaker. In addition, since that loudspeaker is so close and arriving at your listening position early, your brain will be able to figure out that the loudspeaker is close because you’re pretty good at localising sources when they’re near your head. The same problem, albeit on a much smaller scale, happens with the centre loudspeaker. If its time-alignment delay is calibrated using the centre position, then, if you’re sitting on the left side of the sofa, then the Left Front loudspeaker’s signal will arrive before the Centre Front. The end result of this is that, if you’re sitting on the side of the sofa, you’ll have too much from one of the surround loudspeakers and the intelligibility of the dialogue will be reduced a little.
So, how should we calibrate the system to make things a little better for your friends? Take a look at Figure 5, below.
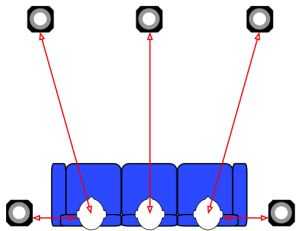
What I’m trying to show with this diagram is that both the distance and the level for each loudspeaker should be measured to the closest person in your listening area. So, in this case, the Left Front and Left Surround loudspeakers are calibrated to the left position on the sofa. However, the Centre Front loudspeaker is calibrated at the centre of the sofa. The result of this is that the centre speaker will be delayed – but less than it would have been if you had calibrated it as in Figure 4, because the Left Front loudspeaker is closer to the person on the left side of the sofa than to the person in the centre of the sofa. Also, the Surround loudspeakers will be delayed much more than they would have been using the scheme in Figure 4. However, they’ll still be symmetrical (so the person in the “sweet spot” won’t feel like the surround channels are lopsided, and the friends on the sides of the sofa won’t notice that they’re sitting on top of a loudspeaker… Also, this will result in the centre channel being a bit louder and the surround channels being a little lower in level – both of which are technically incorrect for the person in the sweet spot, but at least it’s a mistake in the right direction – so you’re improving intelligibility of the dialogue
If you do calibrate the system this way, you’ll technically be incorrectly calibrated at the sweet spot, but your friends on the sides of the sofa will be much happier – and you won’t notice too much. Of course, if you have a BeoPlay V1, a BeoVision 11 or a BeoSystem 4, you can make this configuration just one of your nine available Speaker Groups – you can always use another one for a “perfect” calibration for the sweet spot when you’re home alone.
If, after aligning your system using this method, you still find that the dialogue is a little hard to understand, and the surrounds are a little hot (this is often the case when your sofa and the surround loudspeakers are all situated against the same wall, you should not be afraid to do the following:
If your listening area is larger, the technique is the same – you calibrate any given loudspeaker in the system to the closest listening position, and then tweak to taste.
I guess that the big message here is “just because your system is configured ‘correctly’ doesn’t mean that it can’t sound better”. Don’t be more afraid to tweak the adjustments on your calibration than you would be to add cream and sugar to your coffee, or salt and pepper to your meal in a restaurant. As Duke Ellington once said: “If it sounds good, it is good.”
#13 in a series of articles about the technology behind Bang & Olufsen loudspeakers
The following question recently arrived in my inbox via our customer service department.
“I am an admirer of B&O Hifi products as of over 20 years, but a great mystery for me is how you achieve great sound reproduction in the typically minimalist Scandinavian interior design environmment with polished floors, bare walls and bare glass windows. Effectively such environments are acoustical disasters !?!”
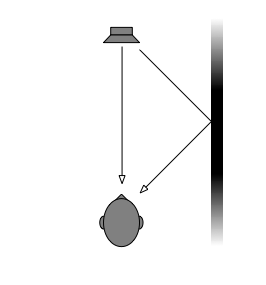




However, there is one interesting effect that sits on top of all of this – that is the fact that what you’ll see in a measurement with a microphone is not necessarily representative of what you’ll hear. This is because a microphone does not have two ears. Also, the direction the reflection comes from will change how you perceive it. A sidewall reflection sounds different from a floor reflection. This is because you have two ears – one on each side of your head. Your brain uses the sidewall reflections (or, more precisely, how they relate to the direct sound) to determine, in part, how far away a sound source is. Also, since, in the case of sidewall reflections, your two ears get two different delay times on the reflection (usually), you get two different comb-filter patterns, where the peaks in one ear can be used to fill in the notches in the other ear and vice versa. When the reflection comes from the floor or ceiling, your two ears get the same artefacts (since your two ears are the same distance to the floor, probably). Consequently, it’s easily noticeable (and it’s been proven using science!) that a floor or ceiling reflection has a bigger timbral effect on a loudspeaker than a lateral (or sideways) reflection.
There are two audible effects of this. The first is that, if your music contains the frequency that the room wants to resonate at, then that note will sound louder. When you hear people talk of “uneven bass” or a “one-note-bass” effect, one of the first suspects to blame is a room mode.
The second is that, since the mode is ringing along with the music, the overall effect will be muddiness. This is particularly true when one bass note causes the room mode to start ringing, and it keeps ringing when the next bass note is playing. For example, if your room rings on a C#, and the bass plays a C# followed by a D – then the room will be ringing at C#, conflicting with the D and resulting in mud. This is also true if the kick drum triggers the room mode, so you have a kick drum “plucking” the room ringing on a C# all through the track. If the tune is in the key of F, then this will not be pretty.
If you would like to calculate a prediction of where you’ll have a problem with a room mode, you can just do the following math:
metric version: room mode frequency in Hz = 172 / (room length in metres)
imperial version: room mode frequency in Hz = 558 / (room length in feet)
Your worst modes will be the frequencies calculated using either of the equations above, and multiples of them (i.e. 2 times the result, 3 times the result, and so on).
So, for example, if your room is 5 m wide, your worst-case modes will be at 172 / 5 = 34.4 Hz, as well as 68.8 Hz, 103.2 Hz and so on. Remember that these are just predictions – but they’ll come pretty close. You should also remember that this assumes that you have completely immovable walls and no absorption – if this is not true, then the mode might not be a problem at all. (If you would like to do a more thorough modal analysis of your listening room, check out this page as a good start.)
Sadly, there is not much you can do about room modes. There are ways to manage them, including, but not exclusive to the following strategies:
Reverberation is what you hear when you clap your hands in a big cathedral. It’s the collection of a lot of reflections bouncing from everywhere as you go through time. When you first clap your hands, you get a couple of reflections that come in separated enough in time that they get their own label – “early reflections”. After that, there are so many reflections coming from so many directions, and so densely packed together in time, that we can’t separate them, so we just call them “reverberation” or “reverb” (although you’ll often hear people call it “echo” which is the wrong word to use for this.
Reverb is what you get when you have a lot of reflective surfaces in your room – but since it’s so irregular in time and space, it just makes a wash of sound rather than a weird comb-filter effect like we saw with a single reflection. So, although it makes things “cloudy” – it’s more like having a fog on your glasses instead of a scratch. Think of it like the soft focus effect that was applied to all attractive alien women on the original Star Trek – you lose the details, but it’s not necessarily a bad thing.
Fine, this is a short-form version of what a room’s acoustics does to the sound of a loudspeaker, but how do we, as a manufacturer of loudspeakers, ensure that our products can withstand the abuse that your listening room will apply to the sound? Well, there are a number of strategies that we use to do what we can…
1. ABC. The BeoLab 5 has a proprietary system built-in called Adaptive Bass Control or ABC. Pressing a button at the top of the loudspeaker starts a measurement procedure that is performed using a built-in microphone that measures the loudspeaker’s behaviour in two locations. Actually, what it’s doing is looking at the difference in the loudspeaker’s response in those two positions of the microphone to determine the radiation resistance that the loudspeaker “sees” as a result of reflective surfaces in the room. The ABC algorithm then creates a filter that is used to “undo” the effects of some of the low-frequency effects of the room’s acoustics. For example, if the radiation resistance indicates that the loudspeaker is close to a wall (which, as we saw above, will boost the bass) then the filter will reduce the bass symmetrically. That way, the loss in the filter and the gain due to the wall will cancel each other.
2. Position switches. ABC in the BeoLab 5 is a very customised filter that, in part, will adjust the loudspeaker’s response for placement near a wall or in a corner. Almost all of the other BeoLab loudspeakers (and other sound systems such as the BeoPlay A8 and A9, for example), include a manual-adjusted “position switch”. This allows you to use one of three filters that we have customised in the development of the loudspeaker to account for its behaviour according to whether you have placed it away from a reflective surface (“Free”), near one surface (“Wall”) or in a Corner. This is not just a filter that adjusts the bass level. The three filters for “free”, “wall”, and “corner” have been calculated using three dimensional measurements of the acoustical behaviour of the loudspeaker. So, the filters for the BeoLab 3 are completely different from those for the A9, for example, because they have very different directivity characteristics.
3. Sound design in multiple rooms. As I talked about in a previous posting, when we do the sound design of all of our loudspeakers, we tune each of them in at least 4 or 5 rooms with very different acoustical behaviours ranging from a very “dead” living room with lots of absorptive and diffusive surfaces to a larger and very “live” space with a minimalistic decorating, and large flat surfaces (just like the description in the original question). Once we have a single sound design that is based on the common elements those rooms, we test the loudspeakers in more rooms to ensure that they’ll behave well under all conditions.
Of course, I haven’t covered everything there is to know about room acoustics here. And, of course, you can’t expect a loudspeaker to sound exactly the same in every room. If that were true, there would be no such thing as a “good”concert hall. A room’s acoustical behaviour affects the sound of all sound sources in the room. On the other hand, humans also have an amazing ability to adapt – in other words you “get used to” the characteristics of your listening room. Back when I was working as a part-time recording engineer in Montreal, I did a lot of recordings in churches. Typically, we (the producer and I) would set up a control room with loudspeakers in a back room, and the musicians would sit out in the church. When we arrived to set up the gear, the first thing was to set up the monitor loudspeakers and a CD player, and we would play CD’s that we knew well while we set up everything else. That way, we would “learn” the characteristics of the control room (since we already knew what was on the discs and the characteristics of the monitor loudspeakers). So, if all of our CD’s sounded like that had too much bass, then we should do a recording with too much bass – it was the fault of the control room.
However, there is no debate that, due to lots of issues (the first two that come to mind are frequency range and directivity) two different loudspeakers will behave differently from each other in two different rooms. In other words, if you listen to loudspeaker “A” and loudspeaker “B” in a showroom of a shop, you might prefer loudspeaker “A” – but if you took them home, you might prefer loudspeaker “B”. This would not be surprising, since what you hear is not only the loudspeaker but the loudspeaker “filtered” by the listening room. This is exactly why, when you are buying a loudspeaker, you should audition it in your home in order to ensure that you will be happy with your purchase. And THIS is why you can arrange a home demonstration of Bang & Olufsen loudspeakers through your dealer.
#12 in a series of articles about the technology behind Bang & Olufsen loudspeakers
As I mentioned in an early posting about two months ago, if I didn’t have time to write enough, I’d cop out and post some photos of development models of some of our loudspeakers. It’s been a busy week. So, here are some photos of the BeoLab 18 and BeoLab 14.






homecinemachoice.com‘s review (March 2014 edition)
“… the power output is phenomenal. Moreover, the detail is incredible and the tonal balance is spot on. The vocals in Antony & The Johnson’ Twilight are intense, the throb of Jeff Beck’s guitar in So Real resonates sublimely, whilst classical works yield profound levels of clarity. The mi-range is highly detailed, the treble is smooth and accurate, and the bass is rich and velvety. You can push the volume without risk: even at high levels the speakers have plenty in reserve.”
#11 in a series of articles about the technology behind Bang & Olufsen loudspeakers
I realised this week that I’ve been throwing around words like ” voice coil”, “suspension”, “surround” and “spider” when I talk about loudspeakers, but many people don’t know what these things are – or how a loudspeaker driver works in general… So, this week, I thought I might back up a couple of steps and talk about some basics. There’s nothing here about Bang & Olufsen loudspeakers specifically – it’s just an introduction to how loudspeaker drivers (like woofers, for example) work.
Back in 1820, a Danish guy named Hans Christian Ørsted was in the middle of giving a lecture when he noticed that, when he switched a circuit on and off, a compass sitting nearby on the desk moved a little. Since he had been poking around with experiments in electricity and magnetism for years, it didn’t take him long to put two and two together and come up with the idea that, when you run electrical current through a wire, you get a magnetic field around it. (Interestingly, not only did Ørsted figure this out (unless you believe that Romagnosi did it), but he also wrote papers on aesthetics – and he was the first person to isolate the element aluminium – and he founded Den Polytekniske Læreanstalt which, today we call the Danish Technical University. So, he had a big influence on B&O in many respects.)
Nowadays, we know that, by putting current through a wire, you produce a magnetic field that has magnetic lines of force that encircle the wire. The direction of the lines of force are dependent on the direction of current, and the extent by which the magnetic lines of force extend away from the wire is dependent on the amount of current.
Depending on whether or not you believe Benjamin Franklin you can use your right or left hand to determine the direction of the lines of force. Figure 1, below, shows a right hand (which indicates that we believe Benjamin Franklin and we say that current runs from the positive terminal of a battery to the negative terminal, which is, in fact, incorrect.). The thumb points in the direction of the current and your other fingers wrap around the wire in the same direction as the magnetic lines of force (which go from North to South on a magnet).
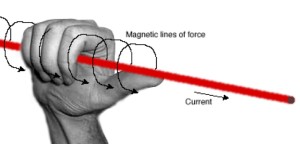
If you take that same wire, keep running current through it, and coil it up like a spring, you can make a slightly more useful magnet that actually has a North pole and a South pole. Again, you can use your right hand to figure out which end is which – you wrap your fingers around the spring in the same direction of the current going through the wire, and your thumb will be pointing towards the North pole of the magnet, as is shown in Figure 2.
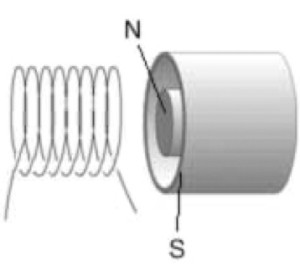
Now, if you take two magnets and you put them end-to-end with the North of one facing the North of the other (or South to South), they’ll push each other apart. If you put them North-to-South, they’ll pull each other together.
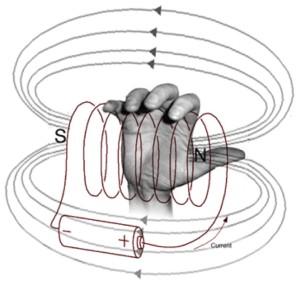
So, let’s do something weird. We’ll make a coil of wire, and we’ll put it in a strange-looking permanent magnet that is a bit like a horseshoe magnet that has been wrapped around itself to make a circular plug in the middle which is one pole (say, North) and a ring around it which is the other pole (say, South) as is shown in Figure 3.
Now, if I put current through the wire, I’ll make a magnetic field around it that will either push against or pull towards the magnetic field of the permanent magnet around it. In other words, if it’s free to move, it will.
Now, since a loudspeaker, generally, is a thing that is used to convert electrical energy into acoustical energy; and, since in order to create acoustical energy (i.e. make noises) we need to move air molecules; we can use this strange device we’ve made in Figure 3 to our advantage. However, let’s be a little more methodical about this…
So, let’s build a dynamic moving coil loudspeaker driver, bit by bit. We’ll start by talking about its name. The “dynamic” part means that the basic principle that does the work is electromagnetism (as opposed to electrostatics or some esoteric methods like using plasma, tesla coils, or cats). The “moving coil” part is because, uh, the part of the device that moves in the magnetic field of the permanent magnet is a coil of wire.
What we want to do is to put the wires of the coil inside a magnetic field that is as strong as we can make it (within reason, of course). The easiest way to do this is to make the “gap” the coil sits in as small as possible (and, of course, to use as strong a magnet as we can fit or lift or afford to buy). So, let’s make a small gap for the coil to sit in.
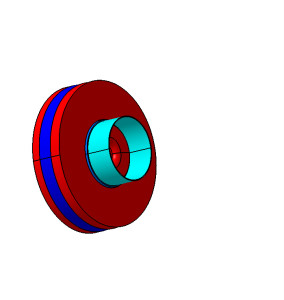
We start by making a “bottom plate” and connect a “pole piece” – this results in a shape that looks like a disc with a cylinder. It’s made out of soft iron because soft iron is a really good magnetic conductor. (In other words, if you stick a magnet on a piece of soft iron, the soft iron basically becomes an extension of the magnet without losing very much magnetic force.) That bottom plate and pole piece assembly is shown in Figure 4, below. I’ve made it red just to keep things clear later. It’s usually not red in real life.
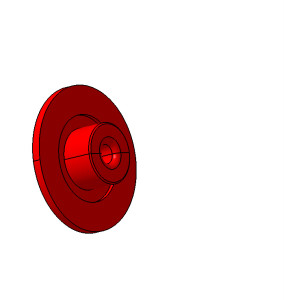
As you can see already, the “plug” in the middle of the magnet in Figure 3 is already visible as part of the pole piece in Figure 4. However, in order to make the strength of the magnetic field greater (in other words, in order to concentrate the magnetic lines of force) we want to make the gap (where the coil is going to sit) narrower. This can be done by making the cylinder on the pole piece a little bigger in diameter – but only where the coil of wire will be. That’s done by putting a ring around it, as is shown by the blue part in Figure 5, below.
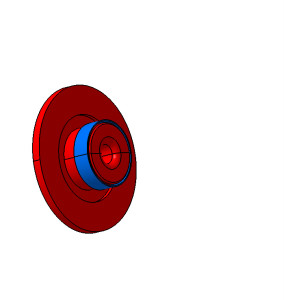
Now we add the magnet as you can see in Figure 6.. This looks like a ring that sits on the disc part of the pole piece. The top of the ring is one pole (say, South) and the bottom is the other pole (say, North) of the magnet. However, this means that the North pole of the magnet is extended to the top of the cylinder on the pole piece because (as I said earlier) the soft iron is a good magnetic conductor.
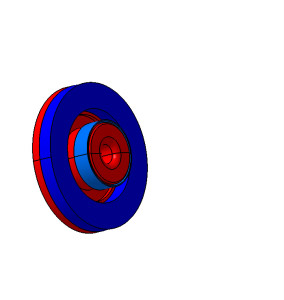
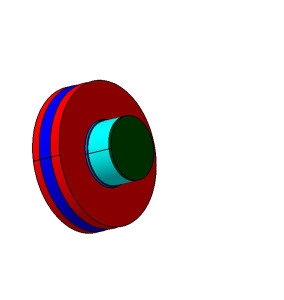
You can see in Figure 6 that the gap between the top of the pole piece and the magnet is pretty big, so let’s make it smaller by putting a “top plate” on the top of the magnet. This is another disc of soft iron, where the hole is just a wee bit bigger than the diameter of the ring around the top of the pole piece as shown in Figure 7. This means that the South pole of the magnet is now the inside edge of the hole in that disc, so we’ve made a circular gap (between the top plate and the ring on the pole piece) that is very small, and therefore has a very concentrated magnetic field.
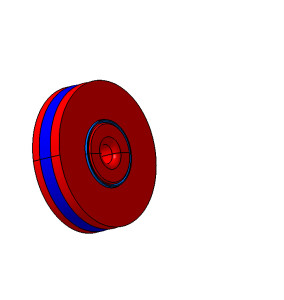
Unfortunately, we can’t just make a coil of wire and stick it in the gap and hope that it’s going to behave. Instead, we take a roll of cardboard (or something else) – a bit like the cardboard tube in the middle of a roll of toilet tissue – and wrap the coil of wire on that. That cardboard roll that supports the coil is called the “former” – it’s shown in Figure 8.
One little extra piece of information here. Since the voice coil, sitting in a magnetic field is the system that essentially converts electrical energy into movement, we call it the loudspeaker driver’s “motor”. Of course, it isn’t a motor that causes something to spin – but it does cause something to move.
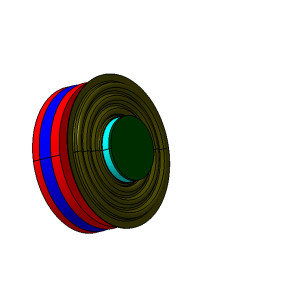
Great. Now we have the coil of wire (the “voice coil”) wrapped around the former, sitting in the magnetic field. So far so good. Now we can put current through the wire and it will want to move in or out of the magnetic (depending on which direction we’re sending the current in). Now, our first problem is that, even if the voice coil and former moved out and in, there is nothing there to push and pull the air molecules in front of it – so it won’t make a lot of noise. So, let’s start putting up a surface that can move some air. We’ll start by putting on a “dust cap” which seals off the end of the former. This is the bump that you see on the front of a woofer in the middle of the cone – so we’re starting to get out to the visible “pretty face” of the loudspeaker driver. The dust cap is shown in Figure 9. Note that the dust cap is not always the same diameter as the former. Note as well that it is usually, but not always convex. Note as well that some drivers don’t have a discrete dust cap (like the BeoLab 3 woofer, for example).
Now we have a problem. We can put current into the coil and get it to move, but there is nothing there to stabilise it. What we need is something to make sure that it doesn’t fall down when you put the loudspeaker on its edge (as most are…). So, we’ll put in a stabiliser. It has to keep the former centred in the magnetic gap, but it also has to be flexible to allow the former to move in and out of the magnet. This part of the loudspeaker is called the “spider” – it looks like a disc that has wiggles in it that can stretch as the former moves in and out. This spider is shown attached to the former in Figure 10. Note that its outside will attached to something else, later.
Welcome to later. Now we need a frame to attach the outside edge of the spider and some other parts of the loudspeaker to – as well as to allow us to attach the whole loudspeaker to a cabinet. This part is called the “basket” – it doesn’t do much other than act as a structural support for everything – a bit like the steel beams in a building. The basket is shown in Figure 11. It may be interesting to note that the basket for an automotive loudspeaker driver is a little different from one for a home loudspeaker because it has to be able to deal with the possibility of a nasty accident. For example, a friend who knows such things once told me that it’s a bad idea to put a woofer intended for a home loudspeaker in a car door because if you’re ever in a side impact collision, it’s not inconceivable that the magnet will rip away from the basket, shoot across the car and cut your legs off. So now I’ve warned you…

Now we can put the rest of the loudspeaker parts on. We attach a “diaphragm” or “cone” which makes the moving surface bigger. That’s the medium-dark green part in Figure 12. If we left it at that, when we moved the loudspeaker in and out of the magnet, it would sag, because the spider isn’t strong enough to keep the whole thing vertical. So, we add a “surround” which is usually made of foam or rubber (or fabric, in the old days). The surround is a flexible ring that is glued to the basket and the edge of the diaphragm. It’s the lightest green thing in Figure 12.
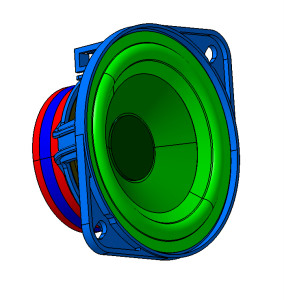
So, now when you put current through the voice coil, it pushes out of (or pulls into) the magnet and moves the former, dust cap and diaphragm with it. This causes the spider and the surround (usually grouped into what we call the “suspension”) to stretch.
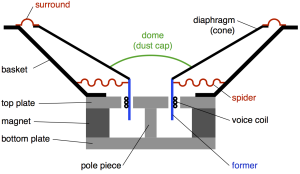
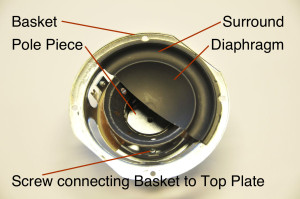
If we take the device in Figure 12 and cut it in half, we get a cross section like the one shown in Figure 13. And, just to prove that I’m not lying, I cut apart a real woofer – it’s shown in Figure 14. And then, not satisfied that I had done enough damage, I did it again to a BeoLab 3 woofer – those photos are in Figures 15 to 19. Another good example is this picture.


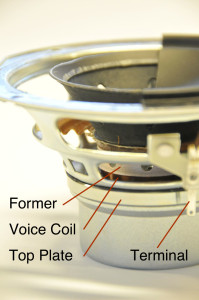
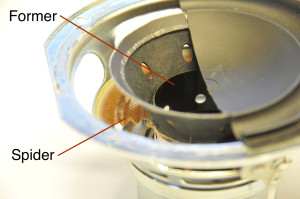
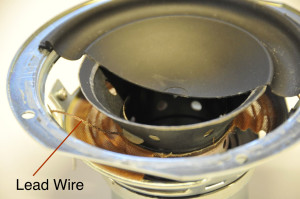
That’s about it for this week. If you want to do a little more digging for yourself, you can look into things like the difference between overhung and underhung voice coils, neodymium vs ferrite, or just watch some relaxing, cool, tangentially-related videos like this one or this one or this one or this one. Or maybe just this.
For the purposes of this explanation, I said that the top of the pole piece is the North pole of the permanent magnet, and the top plate’s inner edge is the South pole. However, there is no fixed convention for this. Manufacturers will almost always ensure that, when you put a positive voltage on the positive terminal of the loudspeaker, the diaphragm will move outwards. However, the north/south-ness of the magnet and the direction the voice coil is wound, and which end of the wire goes to which terminal vary not only from manufacturer to manufacturer, but model to model within one manufacturer’s portfolio.